2understanding The Derivativeap Calculus

1. 2 Understanding The Derivative Ap Calculus Frq
2. 2 Understanding The Derivative Ap Calculus Multiple Choice
3. 2 Understanding The Derivativeap Calculus 14th Edition
4. 2 Understanding The Derivative Ap Calculus Integrals

When you are introduced to the study of calculus in your math class, one of the first concepts you will deal with is derivatives. Derivatives do not sound like your typical math functions that you would be familiar with up this point, but they are a core component of calculus and you will work with them in many ways. In this post, I am going to give you a brief introduction to the concept of the derivative, hopefully answering your question “what is a derivative in calculus,” and then I will follow this up in future posts with more detailed examples of using derivatives.

Consider a curve on a graph – any curve will do. Now, pick a point on the graph, and draw a tangent to the graph at that point. Recall that a tangent to a curve is a line which touches the curve at only a single point. This tangent line is a representation of the derivative of that curve at that particular point on the curve. Naturally, every point on your curve will have its own tangent line. Here is a rough example, where I have generated some curve, and the red lines indicate the tangent lines to the curve at the points where the lines touch the curve:

By using the derivative of the equation of your curve, you are able to precisely calculate what the tangent line will look like at any point on your curve. In fact, the derivative of your equation will very likely be another equation, and you can graph this new equation out to see the graph of the derivative, which itself can be interpreted and used in many ways in calculus. You can even find the derivative of the derivative, etc.

Let’s look at a more practical example to hopefully convey the usefulness and significance of calculating derivatives.

If we consider two points A and B, and we know it takes some time to travel from A to B, we can calculate the “average” velocity of this trip by dividing the distance between the two points by the time taken to travel between them. This should be a familiar notion. However, consider this: if we are driving in a car from home to the market, then we do not have the same velocity for the entire trip. We have to start accelerating from being parked, and then decelerate when we come to stop signs, and then accelerate again, and decelerate to a stop when we reach our destination. You can calculate the average velocity of this trip in the way I mentioned above, but the “instantaneous” velocity at any particular point of the trip might be what you are more interested in. How might you go about doing this? Find the derivative! A graph of our trip, where we plot distance travelled against time, might be useful in this case. We can determine the tangents to this curve at any particular points. We know from previous graphing experience that these tangent lines have a slope of rise over run, and distance over time (the y and x axes on this graph) is velocity. So therefore, the tangent line (specifically, its slope) at any particular point on our curve actually represents the instantaneous velocity at that point. So, we can find out exactly how fast we are going at any point of our trip. We just demonstrated this graphically, but by calculating the derivative of our “trip equation” means that we can calculate how fast we were going any any point without actually graphing our equation at all!

This is just an introduction to the concept of derivatives in calculus, but in future posts, I’ll go into more details so that hopefully you can become more familiar with them. The concept might be new and different, but once you begin working with them, you will see that they are not that difficult to work with at all.

You know you’ll have to know your derivatives inside and out in order to score high on the AP Calculus exam. But what are the most common derivatives you’ll see on the test? In this short article, we’ll let you in on the secret!

Know the Basics

First of all, it’s very important to have the basics down. That includes: the Power Rule, Product Rule, Quotient Rule, and Chain Rule, among others.

June 21, 2015 Math Concepts average velocity, calculus, derivative, instantaneous velocity, what is a derivative, what is a derivative in calculus Numerist-Shaun When you are introduced to the study of calculus in your math class, one of the first concepts you will deal with is derivatives. Check out Calculus Review: Derivative Rules and Derivatives on the AP Calculus AB & BC Exams: A Refresher for more. Common Derivatives. Now let’s take a look at a few problems involving common derivatives that are modeled after actual AP Calculus problems. Find The tangent line to the curve f(x) = x 4 + 3x – 10 at the point (1.

Don’t forget about those special functions either. You’ll have to memorize the derivative rules for trig, exponential, and logarithmic functions.

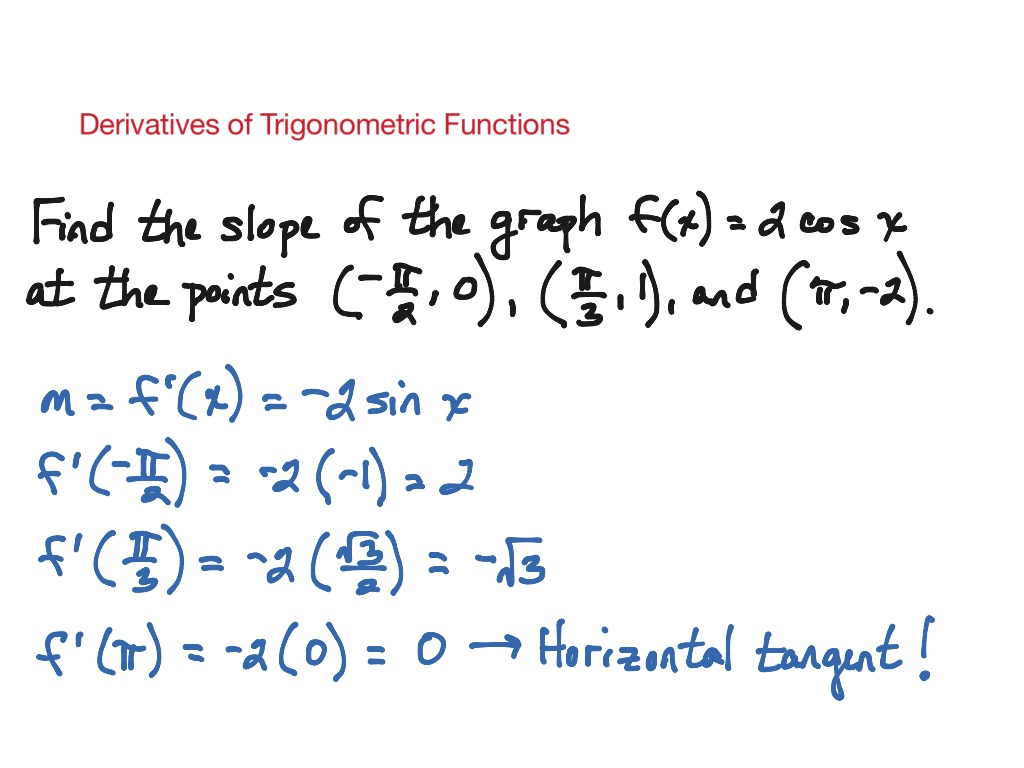
Derivatives of trigonometric, exponential, and logarithmic functions

Less common, but no less important are the rules for inverse trig functions.

Check out Calculus Review: Derivative Rules and Derivatives on the AP Calculus AB & BC Exams: A Refresher for more.

Common Derivatives

Now let’s take a look at a few problems involving common derivatives that are modeled after actual AP Calculus problems.

Problem 1

Find The tangent line to the curve f(x) = x⁴ + 3x – 10 at the point (1, -6).

Solution

To find a tangent line, first take the derivative. Here, we have to use the Power Rule and Sum/Difference Rule.

f '(x) = 4x³ + 3

Then you can find the slope and the equation of the tangent line.

Slope at x = 1: f '(1) = 4(1)³ + 3 = 7

y – (-6) = 7(x – 1)

y = 7x – 13.

Problem 2

The position of a particle moving along the x-axis at time t is x(t) = sin( cos( 4t ) ), for 0 ≤ t ≤ π. Find
the velocity at time t = π/8.

Solution

Find velocity by taking the derivative of the position function. Be careful — we need two applications of the Chain Rule for this one!

v(t) = x '(t) = cos( cos( 4t ) ) ( -sin( 4t ) ) (4)

At time t = π/8, the velocity is equal to:

v(π/8) = cos( cos( 4(π/8) ) ) ( -sin( 4(π/8) ) ) (4) = cos( cos( π/2 ) ) ( -sin( π/2 ) ) (4)

= cos(0)(-1)(4) = (1)(-1)(4) = -4.

Problem 3

Find all inflection points of the curve defined by .

Solution

You can find inflection points by taking the second derivative. Of course, you must take the first derivative first. Don’t forget to rewrite your radical as a power and use Chain Rule.

Use Quotient Rule to help find the second derivative.

After simplification, we find that the second derivative is never equal to 0 and never undefined. Therefore this function has no inflection points.

Problem 4

Solution

2 Understanding The Derivative Ap Calculus Frq

You’ll need both the Product and Chain Rules for this one.

Problem 5

Suppose g(x) = e^4x + 5. At what value of x is the slope of the tangent line to g(x) equal to 3?

Solution

Again, the slope of the tangent line is equal to a derivative value.

g '(x) = 4e^4x

2 Understanding The Derivative Ap Calculus Multiple Choice

Set g '(x) = 3 and solve:

4e^4x = 3

e^4x = 3/4

4x = ln(3/4)

2 Understanding The Derivativeap Calculus 14th Edition

x = ln(3/4) / 4 ≈ -0.0719

Summary

The five problems above represent just a small sampling of what you’ll find on an AP Calculus AB or BC exam. Nevertheless, the majority of problems involving derivatives do tend to fall into these basic formats.

• Know your basic rules, especially the Chain Rule.
• Memorize the derivatives of the special functions.
• And above all, know which tools apply in each situation.

Good luck on the exam!

Improve your SAT or ACT score, guaranteed. Start your 1 Week Free Trial of Magoosh SAT Prep or your 1 Week Free Trial of Magoosh ACT Prep today!

More from Magoosh

About Shaun Ault

Shaun earned his Ph. D. in mathematics from The Ohio State University in 2008 (Go Bucks!!). He received his BA in Mathematics with a minor in computer science from Oberlin College in 2002. In addition, Shaun earned a B. Mus. from the Oberlin Conservatory in the same year, with a major in music composition. Shaun still loves music -- almost as much as math! -- and he (thinks he) can play piano, guitar, and bass. Shaun has taught and tutored students in mathematics for about a decade, and hopes his experience can help you to succeed!

« APUSH Exam Review: 5 Last Minute Test Prep TipsAP Calculus Review: Discontinuities »

Leave a Reply

2 Understanding The Derivative Ap Calculus Integrals

Magoosh blog comment policy: To create the best experience for our readers, we will approve and respond to comments that are relevant to the article, general enough to be helpful to other students, concise, and well-written! :) If your comment was not approved, it likely did not adhere to these guidelines. If you are a Premium Magoosh student and would like more personalized service, you can use the Help tab on the Magoosh dashboard. Thanks!